
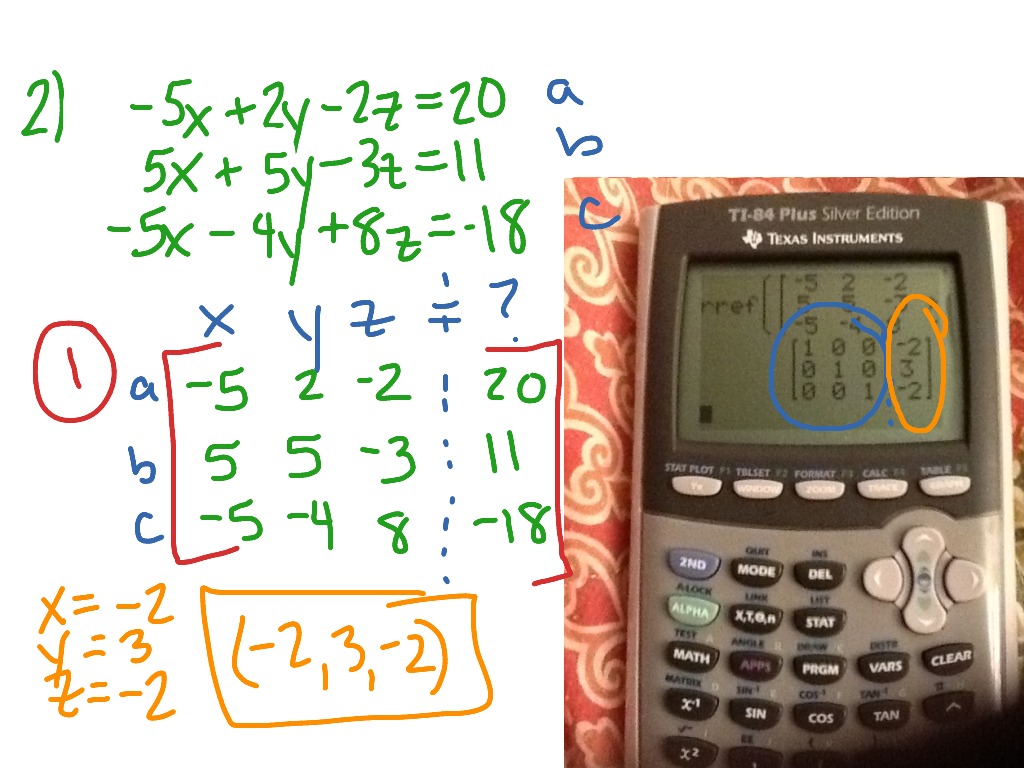
Planes intersect in a line: consistent system called dependent with an infinite number of solutions Remember the solution would be where all three planes all intersect. Graphs of three variable equations are planes. Planes intersect at a point: consistent with one solution With two equations and two variables, the graphs were lines and the solution (if there was one) was where the lines intersected. No solution Three Equations Containing Three Variables As before, the first two cases are called consistent since there are solutions. A dependent system with infinitely many solutions 3. Exactly one solution, an ordered triple (x, y, z) 2. Systems of Linear Equations The solution will be one of three cases: 1.
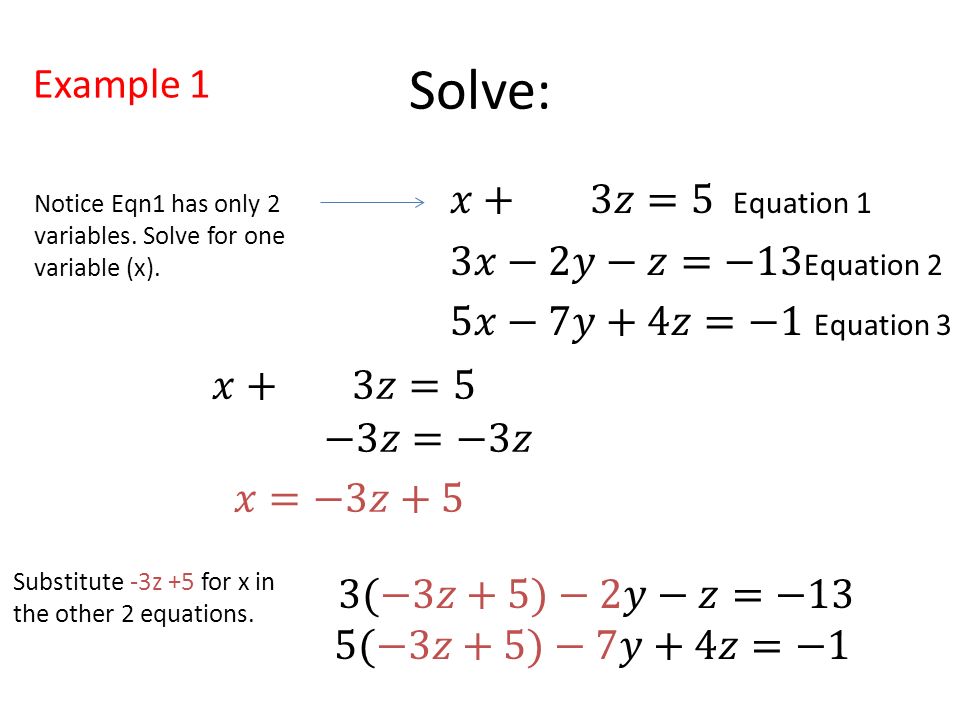
This tells us the system of equations is inconsistent and there is not an x and y that make both equations true. 2 2 This time the y 's were eliminated too but the constants were not. Let's try another one: Let's multiply the first equation by 2 and add to the second to eliminate the x 's. (Solving for x in either equation will give the same result) Any point on this line is a solution So we list the solution as: Let's solve the second equation for x. If y is 2, x is -1 so the point (-1, 2) is a solution to both equations. If y is 0, x is -5 so the point (-5, 0) is a solution to both equations. Now to get a solution, you chose any real number for y and x depends on that choice. This is Case 2 where the lines coincide and all points on the line are solutions. This tells us the equations are dependent. Let's multiply the second equation by 3 and add to the first equation to eliminate the x 's. With either method, we may end up with a surprise. However, if one variable is already or easily solved for, substitution is generally quicker. If substitution would involve messy fractions, it is generally easier to use the elimination method. So we arrived at the same answer with either method, but which method should you use? It depends on the problem. Substitute this for x in one of the equations to find y. (we could instead have multiplied the top equation by -2 and eliminated the x's) Add first equation to this. The idea is to multiply one or both equations by a constant (or constants) so that when you add the two equations together, one of the variables is eliminated. Now let's look at the second method, called the method of elimination. We can check this by subbing in these values for x and y. So our solution is This means that the two lines intersect at this point. Easiest here since we already solved for y. Now we only have the x variable and we solve for it. Substitute this for y in the first equation. You can pick either variable and either equation, but go for the easiest one (getting the y alone in the second equation will not involve fractions). Let's solve for y in the second equation. The idea is to solve for one of the variables in one of the equations and substitute it in for that variable in the other equation. If we have two equations and variables and we want to solve them, graphing would not be very accurate so we will solve algebraically for exact solutions. dependent consistent consistent independent inconsistent Case 3: The lines are parallel so there is no solution. Case 2: The lines coincide and there are infinitely many solutions (all points on the line). Case 1: The lines intersect at a point ( x, y ), the solution. With two equations and two variables, the graphs are lines and the solution (if there is one) is where the lines intersect. No solution Two Equations Containing Two Variables The first two cases are called consistent since there are solutions. Exactly one solution, an ordered pair (x, y) 2. Solve the systems of equation in our example.Solving systems of equations in 3 variables Solve the systems of equations (this example is also shown in our video lesson) When solving systems of equation with three variables we use the elimination method or the substitution method to make a system of two equations in two variables.


 0 kommentar(er)
0 kommentar(er)
